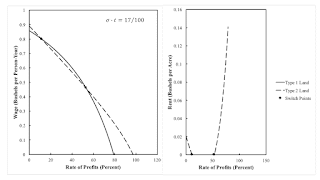
Figure 1: Wage Curves and Rent1.0 Introduction I might as well illustrate an example with extensive rent and reswitching. I find it incredible that the agents in these sorts of models understand the implications of, say, a variation of the distribution of income for their self-interests. Nevertheless, I try to note the consequences of variation in the distribution of income and perturbations of model parameters on prices of production. And I do not worry too much about disequilibria. 2.0 Technology and Requirements for Use Consider a capitalist economy in which two commodities, iron and corn, are produced. One process is known for producing iron. In the iron industry, workers use inputs of iron and corn to produce an output of iron. The output of the iron industry is one ton with the
Topics:
Robert Vienneau considers the following as important: Example in Mathematical Economics, Joint Production, Sraffa Effects
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes Double Fluke Cases For Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes The Emergence of Triple Switching and the Rarity of Reswitching Explained
Robert Vienneau writes Recap For A Triple -Switching Example
| Figure 1: Wage Curves and Rent |
I might as well illustrate an example with extensive rent and reswitching. I find it incredible that the agents in these sorts of models understand the implications of, say, a variation of the distribution of income for their self-interests. Nevertheless, I try to note the consequences of variation in the distribution of income and perturbations of model parameters on prices of production. And I do not worry too much about disequilibria.
2.0 Technology and Requirements for UseConsider a capitalist economy in which two commodities, iron and corn, are produced. One process is known for producing iron. In the iron industry, workers use inputs of iron and corn to produce an output of iron. The output of the iron industry is one ton with the inputs shown in Table 1. Two processes are known for producing corn. Each corn-producing process operates on a specific type of land. The coefficients of production shown in Table 1 are for an output of one bushel corn. These processes can be thought of as examples of joint production. Their outputs are corn and the same quantity of land used as input, unchanged by the production process. Presumably, some of the labor in these processes is used to maintain the land in a given state. For this post, I assume σt is 17/100.
| Input | Iron Industry | Corn Industry | |
| I | II | III | |
| Labor | a0,1 = 1 | a0,2 = 5191/5770 | a0,3 = (305/494) e(3/20) - σt |
| Type 1 Land | 0 | c1,2 = 1 | 0 |
| Type 2 Land | 0 | 0 | c2,3 = e(3/20) - σt |
| Iron | a1,1 = 9/20 | a1,2 = 1/40 | a1,3 = (3/1976) e(3/20) - σt |
| Corn | a2,1 = 2 | a2,2 = 1/10 | a2,3 = (229/494) e(3/20) - σt |
The specification of technology is completed by noting the values of parameters for the quantities available of non-produced means of production. For this numerical example, let there be 100 acres of type 1 land and 100 acres of type 2 land. The iron-producing process and each corn-producing process exhibits constant returns to scale, up to the limits imposed by the endowments of land.
I consider stationary states with a net output consisting solely of corn. A bushel corn is the numeraire. Any one of four techniques can be used to produce corn, depending on the requirements for use. The process for producing iron is part of each technique. Table 2 specifies which types of land are fully or partially farmed in each technique. In the Alpha and Beta techniques, both types of land are cultivated, with one type only partially farmed. In the remaining two techniques, one type of land is left totally farrow. Which techniques are feasible depends on the endowments of the land and on the requirements for use.
| Technique | Type of Land | |
| Type 1 | Type 2 | |
| Alpha | Fully farmed | Partially farmed |
| Beta | Partially farmed | Fully farmed |
| Gamma | Partially farmed | Farrow |
| Delta | Farrow | Partially farmed |
Suppose requirements for use, that is, net output of corn, exceed 55.112 bushels and fall below 80.90. Delta is not feasible. Beta and Gamma are feasible. With Alpha, corn is in excess supply.
2.0 Prices of ProductionI have asserted above that only the Beta and Gamma techniques are feasible, given technology, endowments, and requirements for use. A system of prices of production is associated with each technique. For Beta, type 2 land pays a rent. For Gamma, neither type of land pays a rent.
3.1 Prices for BetaSuppose managers of firms have adopted the Beta technique. Prices of production satisfy the following system of three equations:
(pβa1,1 + a2,1)(1 + r) + wβa0,1 = pβ
(pβa1,2 + a2,2)(1 + r) + wβa0,2 = 1
(pβa1,3 + a2,3)(1 + r) + ρ2c2, 3 + wβa0,3 = 1
In these equations, pβ is the price of iron, wβ is the wage, ρ2 is the rent per acre for type 2 land, and r is the given rate of profits. The left-hand side (LHS) of each equation is the cost of operating the corresponding process at a unit level. Costs include the cost of previously produced commodities used as raw material or ancillary inputs, the going rate of profits on these costs, rent, and wages. Since type 1 land is not fully cultivated, it obtains no rent. The right-hand side (RHS) is the revenue obtained from the corresponding process.
For prices of production, costs do not exceed revenue for any operated process. Furthermore, supernormal profits cannot be made in any prices.
3.2 Prices for GammaNow suppose instead that the Gamma technique is adopted by managers. Prices of production, in analogous notation, must satisfy the following system of equalities and inequalities:
(pγa1,1 + a2,1)(1 + r) + wγa0,1 = pγ
(pγa1,2 + a2,2)(1 + r) + wγa0,2 = 1
(pγa1,3 + a2,3)(1 + r) + wγa0,3 > 13.3 The Choice of Technique
Which system of equations and inequalities prevails for a given rate of profits. The analysis of the choice of technique, in models of extensive rent, can still be based on wage curves. In both the Beta and the Gamma techniques, the first two equations for prices of production are in three variables: the price of iron, the wage, and the rate of profits. Thus, one can solve for the wage as a function of the rate of profits. This is the curve labeled 'Type 1 Land' in the left panel in Figure 1 above.
For the Beta technique, one can solve the last equation for the rent on type 2 land, given the solution from the first two equations. This decomposition of the equations shows that land is a non-basic commodity, in Sraffa's terminology. Hence, a tax on land will not affect the price of iron.
The wage curve for type 2 land can be found from the system of equalities and inequalities for the Delta technique. This wage curve is also shown in Figure 1.
Consider the outer frontier of the wage curves in Figure 1. If requirements for use can satisfied by only cultivating that type of land, then the cost-minimizing technique at a given rate of profits is the corresponding technique. That is, Gamma is cost-minimizing for rates of profits between the switch points.
If the technique for the wage curve on the frontier is not feasible, the corresponding type of land will be fully cultivated. To find the cost-minimizing technique drop down to next wage curve at the given rate of profits. In this example, the cost-minimizing technique corresponds to the wage curve on the inner frontier of the wage curves. So Beta is cost-minimizing at low and high rates of profits. The same rate of profits is made in operating both type 1 and type 2 land, and type 2 land pays a rent.
Whether or not type 2 land is introduced into cultivation alongside partial cultivation of type 1 land depends on the rate of profits. When type 2 land is fully cultivated, less of type 1 land is farmed.
4.0 ConclusionType 1 land is partially farmed. Whether or not type 2 land is fully farmed or left farrow depends on distribution. For high and low rates of profits (or low and high wages), type 2 land is fully farmed and owners of type 1 land receive a rent. For intermediate rates of profits (or wages), type 2 land is left farrow, and no land receives a rent.
Employment is greater under Gamma than when the Beta technique is adopted. Thus, around the switch point at the lower wage, an increased wage is associated with each worker benefitting and employment being increased. Owners of type 2 land have a stake in how the social question is being decided among workers and capitalists.
 Heterodox
Heterodox