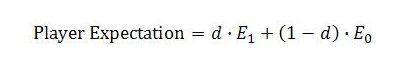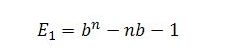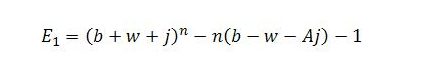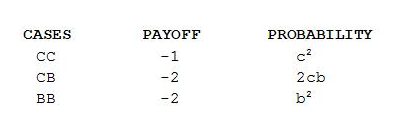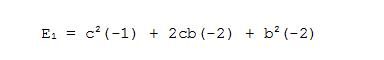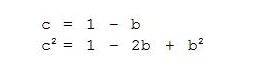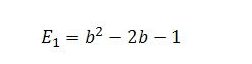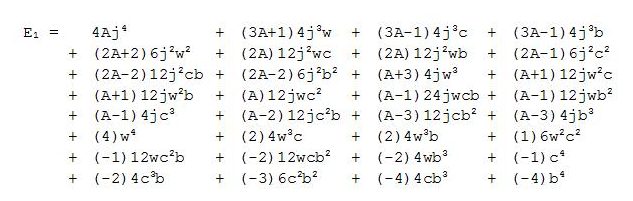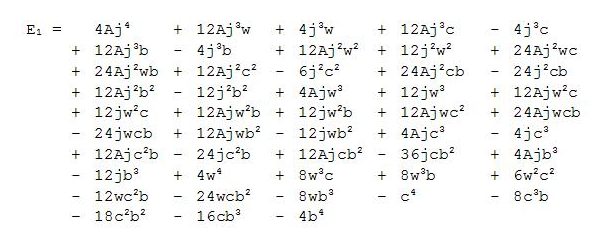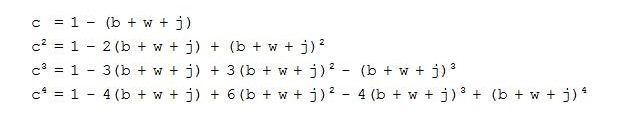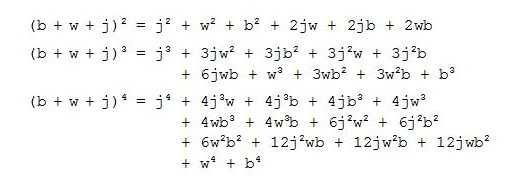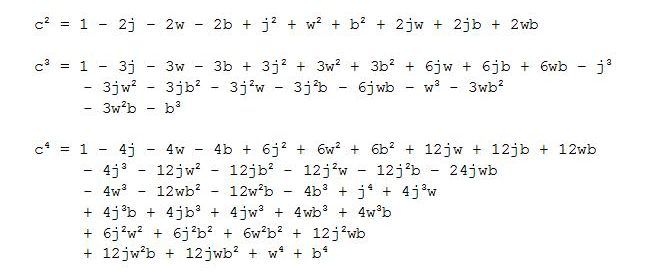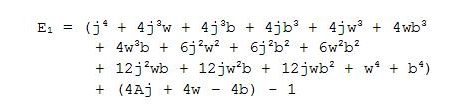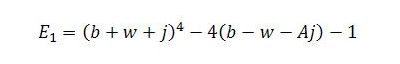Some days, when things seem quiet on the economic front, and even occasionally when they don’t, minds can wander to less weighty matters and become momentarily lost in daydreams of yesteryear; a heady retreat, for instance, back to high school math class one barmy afternoon, sunshine streaming through the windows facing on to Main Quad, with the keener students engrossed in an exploration of the wonders of polynomials; back to a delirious springtime trance interrupted, rudely, by one of the less enthralled students, who wondered aloud, “Yeah, but what good are they in the real world?” Well, it has only been thirty years or so since the more delicate intellects of room 3B were affronted by that question, yet already an example of real-world relevance has surfaced! Imagine this. You are
Topics:
peterc considers the following as important: Other Stuff
This could be interesting, too:
peterc writes Holiday Time 2018
peterc writes Inspector Polynomial in the Strange Case of Busted Bets Plus One
Some days, when things seem quiet on the economic front, and even occasionally when they don’t, minds can wander to less weighty matters and become momentarily lost in daydreams of yesteryear; a heady retreat, for instance, back to high school math class one barmy afternoon, sunshine streaming through the windows facing on to Main Quad, with the keener students engrossed in an exploration of the wonders of polynomials; back to a delirious springtime trance interrupted, rudely, by one of the less enthralled students, who wondered aloud, “Yeah, but what good are they in the real world?”
Well, it has only been thirty years or so since the more delicate intellects of room 3B were affronted by that question, yet already an example of real-world relevance has surfaced!
Imagine this. You are an avid player of blackjack and myriad variations of the game, whether Spanish 21, Australian Pontoon or numerous lesser known offshoots. Perhaps you analyze these games yourself, taking great care, for instance, to generate accurate (if not exact) splitting and re-splitting expectations, which, to put it mildly, is quite a task. Or perhaps you consult highly reputable reference texts, with their bountiful tables of expected values, effects of removal, ingenious strategies and much more. And, finding yourself for some reason in Australia, you are ready to play your favorite game.
What’s that, some of you say? You have two questions … What is splitting a pair? And, for that matter, what is blackjack?
You mean, in trying to imagine that you are a blackjack devotee, you could do with a brief reminder of what the game actually is? Or perhaps you mean that, despite having no inclination ever to break out a pack of playing cards, you are here for the ride: a frivolous foray into one of the lesser known obscurities of the casino world, found in some parts of Australia and possibly nowhere else?
And why not? Curiosity may have killed the cat, but boredom might have done so anyway.
Here is a summary of Australian blackjack in one paragraph. The game is dealt from standard 52-card decks (typically 6 or 8 of them). Aces count as 1 or 11. Kings, Queens, Jacks and Tens count as 10. In the present context, they can just be called ‘Tens’. Other cards take their face values. In the initial deal, each player gets two cards; the dealer only one. A player who receives a Ten and Ace as initial two cards holds a natural 21 (called a ‘natural’ or ‘blackjack’). If the dealer’s first card is neither an Ace nor Ten (so that the dealer cannot achieve blackjack), the player wins immediately and is paid 3 to 2. If the dealer’s initial card is an Ace, the players are given the option to take ‘insurance’, which is a side bet up to half the player’s initial wager (paid at 2 to 1) that the dealer will get a natural. With insurance closed (or never opened), the subsequent deal commences. Players play out their hands first, then if necessary the dealer hand is completed. The player has various options: ‘hit’, ‘stand’, ‘double down’ and ‘split’. Hitting means to draw another card. The player can keep doing this until ready to stand (which means to refuse any more cards) or until the hand busts (the total exceeds 21), in which case the bet is immediately lost. Doubling down involves doubling the initial wager on the condition that only one more card is dealt to the hand. Splitting involves separating a pair (cards of the same rank) into two separate hands. Re-splitting may also be permitted. Each additional split hand requires a separate bet equal to the initial wager. Once players have completed the desired actions on their hands, and so long as there is at least one unresolved player hand, the dealer’s hand is completed. Dealers must follow a fixed strategy that involves only hitting and standing. They must stand on hard totals of 17 or more. Hard totals are those in which all aces (if any) in the hand must count as 1 for the hand not to bust. The dealer must also stand on soft 17 (in some games, soft 18). A soft hand is one in which an ace can count as 11 without busting the hand. At settlement, if the dealer has busted, all non-busted player hands are paid out at 1 to 1. If the dealer successfully makes a hand, player hands with totals closer to 21 are paid 1 to 1, player hands with totals further from 21 lose, and players with the same total as the dealer ‘push’ or ‘standoff’ (a tie). In regular blackjack, a natural beats an ordinary (meaning 3-card or more) 21.
The basis of any casino advantage in the game comes from the fact that if the player busts and then the dealer subsequently busts, the player loses. The blackjack bonus (which only applies to player hands) and the various player options typically enable a player employing perfect basic strategy to reduce the casino’s advantage to somewhere between 0.2 and 0.7 percent, depending on the precise rules and number of decks in use. To gain an advantage, the player needs to employ one or more additional methods in combination with basic strategy. But that is beyond our present scope.
Our major preoccupation, in the present post, concerns what happens when the player splits to multiple hands and the dealer subsequently gets a blackjack. In a typical US casino, this cannot happen, because the dealer initially takes both an up card and a hole card (dealt face down), and checks for possible blackjack before commencing the subsequent deal. If the dealer has blackjack, the round ends immediately and the player loses only the original bet. This rule is referred to as Original Bet Only. In a European casino, the dealer takes an initial up card, but no hole card. A player who splits to multiple hands will lose all bets to a dealer natural. This is known as the European No Hole Card rule. In Australian casinos, the dealer takes no hole card, as in Europe. However, unlike in Europe, the player who splits to multiple hands does not necessarily lose all bets. To appease the kangaroos, it is different. A prominent Australian rule (not the only one, but the one we fixate on here) is referred to as Busted Bets Plus One. Under this rule, if the dealer gets a natural after the player has split pairs, the player loses busted hands plus one more bet if one or more such bets still exist. For example, if the player splits to three hands, doubling one, safely building another hand but busting the third, two bets will be lost, since the player loses the bet on the busted hand plus one more bet. The consequences of this rule are the focus of our investigations!
Okay. So, here you are, back at square one, imagining that you are an avid player of blackjack and myriad variations of the game who just happened to forget what blackjack was there for a moment. You think you’ve seen it all until you sit down at an Australian blackjack table to discover that, for some inexplicable reason, the dealer takes no hole card and, yet, if the dealer winds up with a natural and you have split pairs, you neither lose all bets (as in Europe) nor your Original Bet Only (as in America) but instead Busted Bets Plus One.
You wonder, what is going on with the universe?
Gathering yourself together, and what remain of your gaming chips, you head back to the hotel room to mine your reference books, spreadsheets and algorithms for answers, but no luck. You shoot off an “angry tweet” to your Australian followers, who neglected to divulge this unnerving information upon learning of your intended jaunt to the ends of the earth. This strange casino custom appears to be so obscure that even you have not calculated its significance, despite having calculated just about everything else, including the proportion of out-of-state number plates spotted on the taxi ride from the airport.
Well, there’s no need to fear. You’ve come to the right place. Set that laptop to one side and get out pencil and paper. An eraser and sharpener might also come in handy. Yes, it’s polynomials to the rescue! And a big “I told you they would come in handy” to the doubters of room 3B who pooh-poohed the very idea.
When the dealer shows a Ten or an Ace as up card, the evaluation of splitting can be separated into two parts. One part is the player’s conditional expectation given the dealer achieves a natural, E1, weighted by the probability of this occurrence, d. The other part is the conditional expectation given the dealer does not achieve a natural, E0, again weighted by the corresponding probability, 1 – d.
We need to determine the player splitting and re-splitting expectations given the dealer achieves a natural, denoted E1. Admittedly, this is a much easier task than that of determining E0. The reason for our tight focus is that if the dealer does not have a natural, expectations can be determined in the usual way – and you have an entire wing of a library on that topic, plus a room full of computers back home, and a team of MIT postdocs busily working toward precision to the seventh decimal place. More importantly, E1 can be tackled with polynomials. And that, by any standard, surely trumps all other considerations!
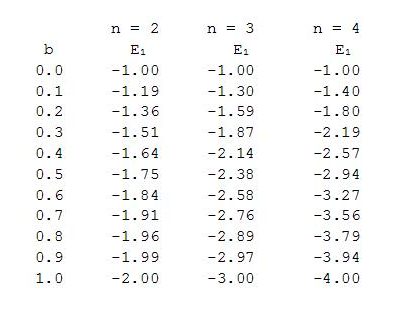
For regular blackjack, in which no split hand beats or matches a dealer natural, it so happens that we only need to know the probability of busting a split hand. If we call this probability of busting a split hand b, and denote the number of split hands as n, it turns out that, at least for n = 2, 3 and 4, the total player expectation (that is, the expectation summed across the n split hands) when the dealer has blackjack can be calculated as:
Derivations of this and the next formula – the fun part! – are left till the next section. If the player never busts (meaning b = 0), then E1 = -1, as in an American game. If the player always busts (b = 1), then E1 = -n, the same as in Europe if no doubling after splitting has taken place.
The following table illustrates how the player’s expectation, conditioned on a dealer blackjack, varies with the bust probability.
Games that are variations on regular blackjack often introduce frightful new rules such as the dealer winning all or some ties. In these games, there will typically be automatic wins or bonus hands to offset some (but usually not all) of the damage to the player.
For such games it is necessary to know the probability of auto wins paid at 1:1, the probability of bonus hands paid at more than 1:1, and the average payoff on bonus hands.
For example, in Australian Pontoon (which, for a basic strategist, is typically a slightly better game than Australian blackjack), an ordinary player 21 wins automatically, paying 1:1, including against a dealer natural; and a player natural, among other hands, pays more than 1:1.
If it is basic strategy to double against dealer Ten or Ace, and doubling after splitting is allowed, the probability of a bonus hand also needs to reflect the likelihood of regular 21 auto wins resulting from doubles. This is because when the dealer has a natural it is as if the player has risked only one bet to win two, in effect being paid 2:1 (assuming the player does not double ‘stiff’ hands – hands that can bust off just one more card – at least other than successfully …).
Let the probability of an auto win paying 1:1 be w, the probability of a bonus hand j, and the average payoff on bonus hands (assuming a 1 unit bet) A. It turns out, at least for n = 2, 3 and 4, that the player expectation against dealer blackjack under the Busted Bets Plus One rule can be calculated as:
When w = j = 0, we have no auto wins or special bonuses and the formula reduces to the one applicable to regular blackjack.
It should be emphasized that the formula gives the conditional expectation of an initial pair when exactly n hands are formed, not the expectation of the initial pair for when P splits are permitted, denoted SPL P. To determine the latter, it is necessary to use the formula for each number of split hands permitted under the rules and then weight these expectations in accordance with their probabilities of occurrence. For example, with SPL 2, the player will usually split once to form two hands, but occasionally twice to form three hands, so the formula must be used once to find the expectation when n = 2, and again to find the expectation when n = 3, with the expectations appropriately weighted.
Inspection of the formula indicates that in the case of special bonuses and auto wins, it is conceivable in a game with sufficiently exotic rules for the player’s expectation against a dealer natural to be better than -1 (or even positive) for some pairs. This is partly because the “Plus One” in Busted Bets Plus One does not apply to automatic winners or bonus hands and partly because the average payoff on bonus hands may be sufficiently large to introduce this possibility.
Polynomials in the Real World, or: Revenge of the Polynomials
The key formulas provided above can be obtained following the same enthralling procedure for all cases, whether the number of split hands is two, three or four, and whether auto wins and bonus hands are, or are not, part of the game. Here, we will work through the simplest case and the most complicated. All intermediate cases can be approached in the same way.
The simplest case – “vanilla blackjack” – is regular blackjack with only two split hands (only one split permitted) and no auto wins or bonus hands payable against a dealer natural; that is, n = 2 and c = j = 0. The most complicated case (“bizarro blackjack”) would be a game that permitted re-splitting to four hands with both auto wins and bonus hands payable against a dealer natural, denoted n = 4, c > 0, j > 0.
Obtaining the relevant formula in each case involves the following steps:
1. List the possible hand types, their associated probabilities of occurrence and their payoffs in the event of a dealer natural. The player expectation given a dealer natural will then be the sum of the products of the probabilities and payoffs.
2. Express the player expectation in terms of b, w, j and A by noting that the probability of a non-busted, losing player hand is c = 1 – (b + w + j) and substituting for c and powers of c as required.
3. Expand all terms, then collect like terms. Many cancel, leaving the desired result.
Two Split Hands, No Auto Wins or Bonuses – Vanilla Blackjack
When the player can only split to two hands and there are no auto wins or bonuses against a dealer natural, there are just two types of player hands, no bust (C) and bust (B), occurring with probabilities c and b, respectively. This gives rise to only three cases, ignoring order of split hands: no bust, one bust, all bust.
The probably of CB is multiplied by 2 because there are two distinguishable orderings, CB and BC, that have equal likelihood. Referring to the table, the conditional expectation is:
In this simplest of cases:
Substituting for c and c2 in our expression for E1, then expanding and simplifying, we arrive at our first exciting result:
Four Split Hands, Auto Wins and Bonuses – Bizarro Blackjack
This case takes effort, which makes all those drills in the school library during lunch break, when the teachers said you should have been out in the playground socializing and developing interpersonal skills, so worthwhile! The expression for E1 expands to 253 giddy terms that then need to be collected and canceled where appropriate. Nevertheless, the logic and math is just as elementary as in the simplest case, and the reward – as we have seen – is a straightforward formula for a situation that could easily come in handy for anyone who happens to visit Australia and wants to analyze for themselves the impacts of its strange casino customs on player expectation rather than take anyone else’s word for it. It’s hard to imagine anything more “real world” than that!
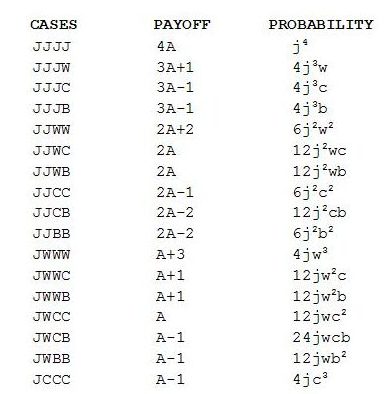
There are now four types of split hands: busted (B), auto winners (W), bonus hands (J) with average payoff A, and non-winning non-busted hands (C), with probabilities b, w, j and c, respectively. There are 35 distinguishable cases – a veritable smorgasbord of delights – shown below. The number of orderings (permutations) of a particular case is equal to n!/(j!w!c!b!). For example, there are 12 ways to order JJWC: 4!/(2!1!1!0!) = 24/(2x1x1x1).
Expressing all this in one marvelous equation:
There are 32 terms at this point, rather than 35, because the three products that involve zero payoffs graciously drop out of the race. Expanding all terms:
At this point, it is useful to note the following:
In these expressions,
These observations enable us to express c2, c3 and c4 as follows:
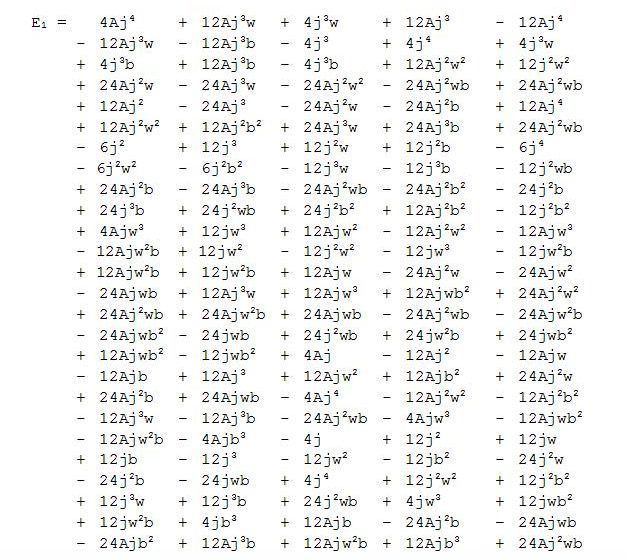
Substituting these expressions into our developing formula for E1 and expanding generates the following wonder:
Obligingly, many terms cancel.
Collecting like terms:
After cancellations, we are left with:
This still looks rather unwieldy and unfamiliar. But by referring back to our earlier notes, a slight sense of panic gives way to a flicker of recognition, and finally relief, that the first bracketed part of this expression for E1 is none other than (b + w + j)4, which entitles us to write our second exciting result:
Beautiful.
Just beautiful.
But … hang on a minute. … That’s all well and good, but what about the various probabilities used in the formula? The b, j and w? Where do they come from?
Well, unless you are especially amazing at mental arithmetic, you will probably be wanting your laptop for that. Useful and highly practical though polynomials are in the real world, they do have their limits.
And besides, from the perspective of polynomials, the problem of determining the b, j and w just does not seem very interesting.
Computers to the Rescue
But no. That would leave things rather incomplete. To put aside the b, j and w would be overly hasty. In the simplest case of the most vanilla of blackjack, we only need worry about b. And not to worry about it would in fact cause us to miss a critical finding!
Now, at first glance, it might seem that our simple formula for n = 2 will have vast applicability. Surely, at the blackjack tables, it will come in to play many times an hour?
Well, if we pause to reflect for a moment, the realization dawns that splitting against a dealer’s Ten (T) or Ace (A) does not occur all that often.
In a typical American game, where the player loses Original Bet Only in the event of a dealer blackjack, it is basic strategy to split A,A and 8,8 against a dealer’s T or A. Under the European No Hole Card rule, where the player loses all bets to a dealer natural, it is basic strategy just to hit these hands, except for A,A v T.
In most respects, the Busted Bets Plus One rule is closer to a holecard game than a European no-holecard game. For this reason, most situations call for the basic strategy that applies when the player loses Original Bet Only. This is clearly correct in the case of doubling, since it is impossible to bust, assuming we don’t do anything suicidal like doubling down on a stiff hand (which most Australian casinos do not permit, in any case). It is also clearly correct in the case of splitting Aces, since in most regular blackjack games, the player only receives one card to each split Ace and so cannot bust.
Therefore, for all our efforts devising a whiz bang formula, the real world applicability of our formula, so far as vanilla blackjack goes, appears to be confined to an analysis of the (heavily losing) situations 8,8 v T and 8,8 v A.
If you are momentarily underwhelmed by this limited applicability, you are forgiven. But we are about to make a “discovery” that few – if any – books on the subject disclose (not because they give incorrect advice but because most blackjack books are not specifically about Australia, which may be the only place where this unusual situation arises).
In what follows, it is assumed that 6 decks are used, the dealer stands on all 17s (soft and hard) and doubling after splitting is permitted.
The most striking case is 8,8 v T.
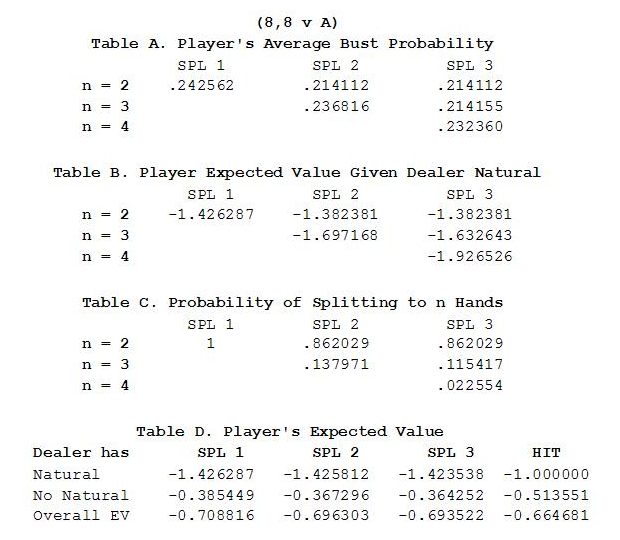
In general, including for split hands, it is basic strategy to hit against a dealer T until the player has at least a standing total of 17. If a 3 is drawn to a split 8, it is basic strategy to double down. Following this strategy on each split hand starting from an 8, the player’s bust probability (b) varies slightly depending on how many split hands (n) are formed and the maximum number of split hands that are permitted SPL P:
These numbers, as already indicated, were not derived through the majesty of the pencil-and-paper polynomials, but rather through the more hard-nosed procedures of the computer. There is a subtle reason why the bust probability for n = 2 hands varies with the rules. When only one split is permitted (SPL 1), each split hand formed with an 8 can receive another 8 as first hit card. This is not true of n = 2 split hands when re-splitting is permitted. Under re-splitting, if an 8 is drawn as first hit card to the first or second split 8, this 8 will itself be split off to form a new hand, and we will be dealing with either n = 3 hands or n = 4 hands.
For a similar reason, there is a difference in bust probability for n = 3 hands, depending on whether 2 or 3 splits are permitted.
Applying our formula (E1 = bn – nb – 1), we get for the various cases:
We also need to weight these expected values in accordance with the frequencies of forming 2, 3 or 4 hands.
Under SPL 1, only one split is permitted, so the probability of forming two hands (n = 2) is one. This and other probabilities are shown below:
The player’s expected value given the dealer has a natural (E1) can now be calculated by multiplying each entry in Table B by the corresponding entry in Table C. The results are shown in the top row of Table D. The second row of Table D shows the player’s expected value when the dealer does not have a natural. These numbers are obtained from the third edition of Don Schlesinger’s Blackjack Attack. The third row of Table D shows the player’s overall expectation when holding 8,8 v T. Each entry in the third row is the weighted average of the two entries directly above it. For example, when the player splits to three hands, three 8s and the dealer’s Ten are removed from six decks, so the probability of the dealer drawing an Ace for blackjack is 24/308, and the probability of no dealer blackjack is one minus this fraction. In addition to the splitting expectations, a fourth column shows the player’s expected value from hitting rather than splitting.
 Our striking result is in the bottom row, far-right column: that bottom figure in the HIT column. It is a result that surely vindicates the polynomials, bringing to light a real-world relevance of stunning proportions!
Our striking result is in the bottom row, far-right column: that bottom figure in the HIT column. It is a result that surely vindicates the polynomials, bringing to light a real-world relevance of stunning proportions!
Yes, it is apparently true. When playing a no-holecard game in which the player loses Busted Bets Plus One to a dealer natural, the correct basic strategy when encountering 8,8 v T is not to split, but rather to hit!
Although the player can improve the splitting expectations conditioned on a dealer blackjack by standing sooner (say on hard totals of 15 or above), the player’s improvement in bust probability does not make up for the cost of this inferior hitting strategy when the dealer does not end up with blackjack.
The savings on a $100 wager for a player who hits rather than splits 8,8 v T, under the Busted Bets Plus One rule, amount to a princely sum ranging anywhere from $8.08 to $8.93, depending on the precise rules in force. We will still lose the equivalent of a $68 shirt off our collective back, but not a $77 shirt.
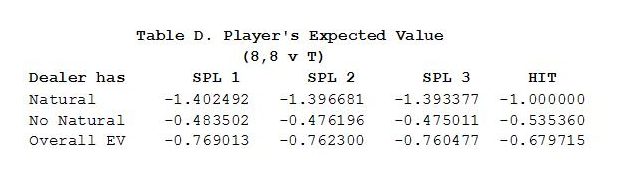
A consideration of 8,8 v A leads us to a similar conclusion: basic strategy is to hit, not split.
The savings in the case of 8,8 v A are smaller. The equivalent tables to those provided in the case of 8,8 v T are shown below without further comment. We actually have more exciting explorations ahead, in the dizzying world of bizarro blackjack.
Computers Love Bizarro Blackjack
Over the last decade or so, there has been a proliferation of games that are variations on regular blackjack, with rules that can appear new and exciting but usually make the game significantly worse for the basic strategist.
To take a specific example, there is a low-stakes game in one Australian casino that pays 2 to 1 on a player blackjack as well as awarding automatic wins for 5-card non-busted hands and ordinary 21s, including against a dealer blackjack. In fact, if the dealer gets a blackjack, any player that happens also to have a blackjack gets paid not at 2 to 1 but at either 3, 4 or 5 to 1, depending on whether the player’s ten-valued card is of lower, the same or higher rank than the dealer’s ten-valued card. This means that, against a dealer blackjack, a player blackjack gets paid, on average, 4 to 1. The player can also double on the first two or three (rather than just two) cards, although there is no redoubling. This sounds great, and sounds even better when it is learned that, unlike in regular blackjack, the player can achieve a natural after splitting Aces or Tens, and in doing so receive the same payouts as already described. In addition, split Aces can be re-hit with the possibility of each hand turning into a 5-card-non-busted or ordinary-21 auto winner.
Not surprisingly, there is a catch. The benefits of these enticing rules are more than negated by the unfortunate circumstance that the dealer wins on all tied totals of 17 to 20. This is very costly, and more than wipes out the benefits of the other favorable rules.
As a consequence, the game has a much worse expectation for the basic strategist than regular blackjack or Australian Pontoon. Worse still, most players will have little idea of correct basic strategy in this and other custom-made versions of the game. In the case of regular blackjack – which is played worldwide – there are many good books published on the subject, with a fair proportion presenting a correct basic strategy (or very close to it). Unfortunately for the casual player, the same resources are not available when it comes to the numerous offshoots of the game. And the more exotic the rules, the more costly it is likely to be if a player incorrectly uses the basic strategy that applies in regular blackjack.
Even so, and awful as these games can be for the player, from a purely analytical viewpoint, some of the quirks can be rather interesting. And that is more than enough to be getting on with! Who has time to play card games, anyway, when these blackjack offshoots throw up so many beguiling mathematical conundrums to explore?
An example is a player who holds a pair of Aces against a dealer Ace. Now, in regular blackjack, the player’s expectation conditioned on no dealer natural is a healthy +0.248335 if the player is permitted to split up to four hands. (The source of this number is once again Blackjack Attack.) But if the dealer draws a Ten as second card for blackjack, the player will lose 1 bet. Since only one card is dealt to each ace, busting is impossible, so the Busted Bets Plus One rule inflicts no additional damage on the player. Overall, in regular blackjack, the player’s expectation with A,A v A is -0.139500; so, a losing situation, all things considered.
This is not so in the version of 2 to 1 blackjack just described. Although most hands in this game are terrible losers for the player, it is quite a different story in the case of A,A v A!
With this one particular, pretty rare hand, the basic strategist’s expected value conditioned on a dealer natural is +1.433937. Yes, you read that right. The player will have a 143 percent advantage, given no dealer blackjack. The more interesting part, though, is that the player’s prognosis actually improves when the dealer does get a blackjack. This is partly because, on average, drawing a Ten to one or more split Aces (up to three split hands can be formed) will be paid 4 to 1. It is also because any 5-card non-busted hands (which are at their most likely for hands starting from an Ace) and ordinary 21s will be auto winners. The upshot is that if the dealer, starting from an Ace, does end up with a natural, the player’s expected value from splitting A,A improves to +2.155747. Overall, the player’s expectation for splitting A,A v A is +1.658285.
The probabilities for bonus hands, auto wins and busts are, regrettably, generated on the computer rather than by pencil and paper with polynomials.
Armed with our much sought-after values for j, w and b, the full power of the polynomials can now be brought into the light. We calculate E1 separately for n = 2 hands and n = 3 hands, applying our formula, E1 = (b + w + j)n – n(b – w – Aj) – 1. This produces the following results:
These values for E1 can now be weighted by the probabilities displayed beside them to give the first entry in our final table. The player EV for when the dealer does not have a blackjack, shown in the second row, is obtained by asking the computer nicely. Weighting the two conditional EVs by their respective probabilities of occurrence gives the overall EV in the bottom row.
Naysayers will reiterate that the analytically interesting aspects of this and other exotic varieties of blackjack do not at all make for a player-friendly game. They will justly insist that almost everyone should avoid such games unless they at least know the correct basic strategy and what the cost of an hour’s play is likely to be, and are happy to incur that expected cost and, in the short run, quite possibly much worse.
But all that pales into insignificance when compared with the complete and utter vindication of the polynomials and their practical application to everyday life.
Related Reading
A methodology for evaluating splitting and re-splitting is outlined in Chapter Eleven of:
Griffin, P. A., 1999, The Theory of Blackjack, Huntington Press, Las Vegas.
Internet poster MGP provides a series of short posts on calculating exact splitting and re-splitting expected values for a fixed (zero memory) strategy taking account only of the player’s pair cards and dealer’s up card. His posts explain how to make repeated use of the conditional probability equation so that SPL 1 can be analyzed with explicit reference to only one shoe state; SPL 2, only three shoe states; and SPL 3, only five shoe states.
MGP’s insight was applied in generating the b, j and w needed for the Busted Bets Plus One formulas discussed in the present post, as well as for analyzing splitting and re-splitting in the variant of bizarro blackjack considered.
For regular blackjack, extensive and accurate tables of basic strategy expectations for different numbers of decks and dealer standing rules, generated by the analyst Cacarulo, are provided in a book already cited twice in the post:
Schlesinger, D., 2005, Blackjack Attack, RGE Publishing, Nevada.