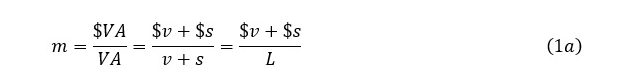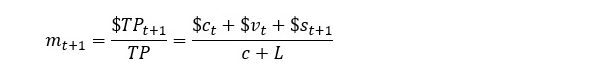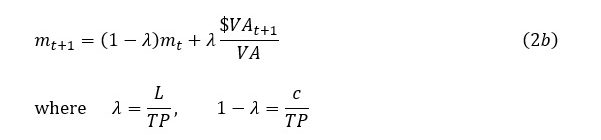In an earlier post it is suggested that when value is conceived as socially necessary labor time, it makes sense to define currency value in one of two ways, either as the reciprocal of the average money wage paid for an hour of simple labor or, alternatively, as the reciprocal of the ‘monetary expression of labor time’ (MELT). Under the first definition, currency value is the amount of simple labor-power commanded by a unit of the currency and, on average, the amount of simple labor that will be performed in exchange for a unit of the currency when advanced as wages. Under the second definition, currency value is the amount of simple labor represented in a unit of the currency; that is, the labor-time equivalent of a currency unit. Currency value in terms of socially necessary
Topics:
peterc considers the following as important: Economics
This could be interesting, too:
Lars Pålsson Syll writes Schuldenbremse bye bye
Lars Pålsson Syll writes What’s wrong with economics — a primer
Lars Pålsson Syll writes Krigskeynesianismens återkomst
Lars Pålsson Syll writes Finding Eigenvalues and Eigenvectors (student stuff)
In an earlier post it is suggested that when value is conceived as socially necessary labor time, it makes sense to define currency value in one of two ways, either as the reciprocal of the average money wage paid for an hour of simple labor or, alternatively, as the reciprocal of the ‘monetary expression of labor time’ (MELT). Under the first definition, currency value is the amount of simple labor-power commanded by a unit of the currency and, on average, the amount of simple labor that will be performed in exchange for a unit of the currency when advanced as wages. Under the second definition, currency value is the amount of simple labor represented in a unit of the currency; that is, the labor-time equivalent of a currency unit.
Currency value in terms of socially necessary labor
Suppose, on average, that workers are paid $15 for an hour of simple labor or its equivalent and in that hour create $30 of value. The money wage is $15/hour. If, for the purposes of the example, productivity is held constant, the MELT (i.e. the monetary value corresponding to an hour of socially necessary labor) is $30/hour. Currency value under the first definition is 1/15 hours (or four minutes) of socially necessary labor per dollar and, under the second definition, 1/30 hours (or two minutes) of socially necessary labor per dollar.
The two measures of currency value differ because workers do not receive the full value generated in production. More generally, the two measures can also differ because of variations in productivity, which raise or lower the bar on what counts as socially necessary labor. When productivity changes, the elements of constant capital, which are the result of past (or ‘dead’) labor, must be re-evaluated. This re-evaluation of constant capital directly affects the MELT, and its reciprocal, but does not directly affect the money wage.
Either measure of currency value can be squared with the approach of Modern Monetary Theory (MMT) which, in general terms, defines the value of the currency as “what must be done to obtain it”. In answer to MMT’s question, “what must be done to obtain the currency?”, the first measure answers that, on average, the equivalent of four minutes of simple labor must be performed in exchange for a dollar received in wages, whereas the second measure answers that, on average, the equivalent of two minutes of value generated in production and represented in commodities must be supplied in exchange for a dollar.
The second definition of currency value can be viewed in terms of a commodity theory. Under a commodity theory, the value of the currency is the amount of labor needed to reproduce a currency-unit’s worth of the ‘money commodity’. During the gold standard era, the value of the currency, from this perspective, would have been the amount of simple labor needed to produce a currency unit’s worth of gold. Previously I have argued that, under capitalism, a suitable candidate for the ‘money commodity’ is actually the commodity labor-power. Currency value can then be regarded as the amount of simple labor needed to reproduce a currency unit’s worth of simple labor-power. If the wage is at the cultural subsistence level, which is the tendency according to Marx, the amount of labor needed to reproduce a currency unit’s worth of labor-power is simply the reciprocal of the MELT, and so corresponds to currency value under the second definition. More generally, allowing for deviations of the wage from the cultural subsistence level, the amount of labor needed to reproduce a currency unit’s worth of labor-power is the value to which the reciprocal of the MELT (or currency value) tends.
To see the connection between the reciprocal of the MELT and the amount of labor needed to reproduce a currency-unit’s worth of labor-power, reconsider the example in which workers are paid $15 for an hour of simple labor or its equivalent and in that hour create $30 of value. Under these circumstances, it takes half an hour (or 30 minutes) to generate $15 of value, which, if the wage reflects cultural subsistence requirements, is the amount sufficient for the reproduction of one hour of simple labor-power. Since it takes 30 minutes to reproduce 15 dollars’ worth of labor-power, it takes 2 minutes (30 minutes divided by 15) to reproduce a dollar’s worth of labor-power, implying currency value of 1/30 hours of socially necessary labor per dollar.
An implementation of MMT’s proposed job guarantee would, in effect, formalize a ‘labor-power standard’ in which labor-power serves as the ‘money commodity’. The policy-administered job-guarantee wage would define a fixed (though policy-adjustable) rate at which simple labor-power is convertible into the currency, on demand. In the absence of a job guarantee – the norm today – the currency lacks a nominal anchor, as Modern Monetary Theorists repeatedly emphasize. This leads to the highly unsatisfactory (and usually tacit) resort by policymakers to a buffer stock of the unemployed (or ‘reserve army of labor’). In a fiat monetary system permitting market exchange, either a buffer stock of the unemployed or a buffer stock of the employed (made possible by the job guarantee) is necessary to contain variations in the value of the currency within tolerable limits.
To summarize the argument to this point, let w represent the average money wage paid for an hour of simple labor and let m represent the MELT. Currency value under the first definition (labor commanded) is 1/w hours of simple labor per dollar. Currency value under the second definition (compatible with a commodity theory) is 1/m hours of simple labor per dollar. Since the money wage is not directly affected by variations in productivity, distribution, or the organic composition of capital, the calculation of currency value under the first definition is the same under all circumstances. In contrast, as proponents of the temporal single-system interpretation of Marx’s theory of value have shown, the method of calculating the MELT (and therefore its reciprocal, 1/m) differs according to whether productivity is constant or variable. The first definition of currency value has the virtue of simplicity, though is somewhat limited in scope. The second definition is more broadly encompassing but introduces complications. Because of the complications (and only for this reason), the remainder of the discussion focuses on the second definition. (I am personally indifferent between the two definitions.)
Currency value as the reciprocal of the MELT – constant productivity
The simplest scenario is when productivity remains constant. In that case the MELT can be calculated as
where VA is value added, v variable capital, s surplus value, and L total productive employment, all measured in hours of simple (socially necessary) labor with quantities of complex labor converted into equivalent amounts of simple labor. A dollar ($) sign indicates a marxian value category measured in monetary terms. As the formula indicates, when productivity is held constant, the MELT is simply monetary value added divided by total productive employment.
In price terms, an equivalent formula is
where P is an index serving as a measure of the price level and Y is total income net of depreciation evaluated at constant prices (a measure of ‘real’ income). In this form, the MELT is nominal net income, PY, divided by total productive employment. Noting that Y/L is average productivity, y,
When productivity is held constant, the MELT can be calculated as the product of the price level and average productivity.
Expression (1c) disguises that, under constant productivity, the MELT is actually independent of average productivity. This can be made explicit by decomposing the price level P. By identity,
where k is one plus the aggregate markup over total money wages and w, as before, is the average wage paid for an hour of simple labor-power. When this expression for the price level is substituted into (1c), productivity appears in both the numerator and the denominator, and so cancels, leaving the MELT independent of average productivity in the constant-productivity case:
Under the simplifying assumption of constant productivity, the MELT is just the product of (one plus) the markup and the money wage, with variations in the MELT reflecting variations in the markup and money wage.
When currency value is defined as the reciprocal of the MELT,
The currency’s value, z, moves inversely to the markup and money wage.
Currency value as the reciprocal of the MELT – variable productivity
The situation is somewhat more complicated once productivity is free to vary because it is no longer valid to confine attention solely to value added (variable capital v plus surplus value s) in the measurement of the MELT. It becomes necessary also to take account of constant capital c. As already anticipated, the reason for this is that changes in productivity in the current period alter the value of inputs produced in earlier periods and so affect the amount of value that is transferred from inputs to newly produced commodities, per unit of output. Since the MELT expresses the monetary value of an hour of socially necessary labor, and since the bar for what counts as socially necessary labor rises whenever productivity improves, the effects of variations in productivity need to be reflected in the calculation of the MELT. More specifically, the devaluing effect of rising productivity on constant capital needs to be taken into account.
Rather than confining attention to value added, in the context of changing productivity the MELT must be related to ‘total price’ (equal, by identity, to ‘total value’). Total price is the sum of constant capital, variable capital and surplus value, and can be measured either in labor-time terms (TP) or monetary terms ($TP). The MELT of time t+1 is identically equal to total price measured in monetary terms divided by total price measured in labor time:
As before, c is constant capital and L (equal to variable capital v plus surplus value s) is total productive employment measured in hours of simple labor.
The current period is assumed to begin at time t and end at time t+1. In the expression for the MELT of time t+1, time subscripts for labor-time variables are suppressed. These variables could have the subscript t,t+1 to indicate that they relate to a labor process that extends in time from the beginning to end of the period. Although the subscripts are absent, the intended meaning is still of an activity that is measured in time. In contrast, it is assumed that monetary flows are evaluated either at the beginning or at the end of the period. By assumption, the monetary advances of constant and variable capital are evaluated (and already known) at time t, when production begins, whereas the monetary measure of surplus value ($st+1) is unknown until the period’s end at time t+1. For a given monetary advance of variable capital at time t, and a given level of employment L, measured in hours of simple labor, a change in productivity over the period will alter the rate of exploitation, s/v, and therefore the distribution of value added between v and s. Since $v is set at time t, any change in s/v can only be reflected in monetary terms through a change in $s.
Marx maintained that an hour of socially necessary labor (i.e. an hour of simple labor) always creates the same value in real terms, at the time it is performed, irrespective of variations in productivity. This means that a given total employment L, measured in hours of simple labor, will always create the same value v+s. So, while the distribution of value added between v and s can change whenever productivity changes, the sum of v and s remains the same so long as the level of total employment measured in hours of simple labor remains the same.
The preceding expression for the MELT of time t+1 is not yet ready for calculation purposes. There is a need to specify the real (labor-time) measure of constant capital c that appears in the denominator. This measure can be obtained by dividing the monetary value of constant capital by the MELT of time t. Making this alteration,
This formula, discussed previously, is due to Ramos-Martinez (the relevant reference can be found at the link). Apart from aiding calculation, the formula makes clear that the MELT of time t+1 (the end-of-period MELT or ‘output MELT’) depends, in part, on the MELT of time t (the start-of-period MELT or ‘input MELT’). The start-of-period MELT is a product of history that enters as a datum into the determination of the end-of-period MELT. If the start-of-period MELT is unknown, it can be approximated to a desired degree of accuracy using a procedure outlined in an earlier post.
It can be shown (see the previous two links) that
Here, $VAt+1 = $vt + $st+1. Expression (2b) says that the end-of-period MELT is a weighted average of the start-of-period MELT, mt, and a measure akin, apart from the temporal aspect, to the constant-productivity MELT, $VA/VA, presented earlier. The weights (λ and 1–λ) are the proportions of total price (and total value) that are attributable to dead and living labor. During production, a fraction c/TP of total value is pre-existing and merely transferred from inputs to the final product, while the remaining fraction L/TP is value that is created through the expenditure of labor-power.
The preceding expression implies that the MELT of time t+1 is also closely connected to the markup and money wage
So, just as in the constant-productivity case, the behavior of the MELT in a context of changing productivity reflects variations in the money wage and markup. As a practical matter, since kw = Py, an alternative means of analyzing the behavior of the MELT is to track the behavior over time of the price level and average productivity. But, unlike in the constant-productivity case, variations in the MELT will also reflect changes in the proportions in which dead and living labor enter total price (the weights, c/TP and L/TP).
Currency value, under the second definition, will of course simply mirror the reactions of the MELT to variations in the money wage, markup, and proportions in which dead and living labor enter total price.