Heterodox
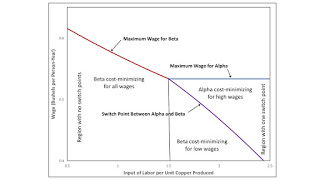
Figure 1: Bifurcation Diagram1.0 Introduction In a series of posts, I have been exploring structural economic dynamics. Innovation reduces coefficients of production. Such reductions can vary the number and sequence of switch points on the wage frontier. I call such a variation a bifurcation. And I think such bifurcations, at least if only one coefficient decreases, fall into a small number of normal forms. One possibility is that a decrease in a coefficient of production results in a switch point appearing over the wage axis, as illustrated here. This post modifies that example such that the switch point disappears over the wage axis with a decrease in a coefficient of production. 2.0 Technology Accordingly, consider the technology illustrated in Table 1. The managers of firms
Topics:
Robert Vienneau considers the following as important: Example in Mathematical Economics, Labor Markets, Sraffa Effects
This could be interesting, too:
Robert Vienneau writes The Production Of Commodities And The Structure Of Production: An Example
Robert Vienneau writes A Derivation Of Prices Of Production With Linear Programming
Robert Vienneau writes Reswitching Pattern In Corn-Tractor Model
Robert Vienneau writes Goal: Perturb Special Case Of Steedman’s Corn-Tractor Model
|
| Figure 1: Bifurcation Diagram |
In a series of posts, I have been exploring structural economic dynamics. Innovation reduces coefficients of production. Such reductions can vary the number and sequence of switch points on the wage frontier. I call such a variation a bifurcation. And I think such bifurcations, at least if only one coefficient decreases, fall into a small number of normal forms.
One possibility is that a decrease in a coefficient of production results in a switch point appearing over the wage axis, as illustrated here. This post modifies that example such that the switch point disappears over the wage axis with a decrease in a coefficient of production.
2.0 TechnologyAccordingly, consider the technology illustrated in Table 1. The managers of firms know of four processes of production. And these processes exhibit Constant Returns to Scale. The column for the iron industry specifies the inputs needed to produce a ton of iron. The column for the copper industry likewise specifies the inputs needed to produce a ton of copper. In this post, I consider how variations in the parameter u affect the number of switch points. Two processes are known for producing corn, and their coefficients of production are specified in the last two columns in the table. Each process is assumed to require a year to complete and uses up all of its commodity inputs.
| Input | Industry | |||
| Iron | Copper | Corn | ||
| Alpha | Beta | |||
| Labor | 21/8 | u | 1 | 3/2 |
| Iron | 1/4 | 0 | 1/4 | 0 |
| Copper | 0 | 1/5 | 0 | 1/5 |
| Corn | 0 | 0 | 0 | 0 |
This technology presents a problem of the choice of technique. The Alpha technique consists of the iron-producing process and the corn-producing process labeled Alpha. Similarly, the Beta technique consists of the copper-producing process and the corn-producing process labeled Beta.
The choice of technique is based on cost-minimization. Consider prices of production, which are stationary prices that allow the economy to be reproduced. A wage curve, showing a tradeoff between wages and the rate of profits, is associated with each technique. In drawing such a curve, I assume that a bushel corn is the numeraire and that labor is advanced. Hence, wages are paid out of the surplus product at the end of the year. The chosen technique, for, say, a given wage, maximizes the rate of profits. The wage curve for the cost-minizing technique at the given wage lies on the outer frontier formed from all wage curves.
3.0 ResultsConsider variations in u, the input of labor in the copper industry, per unit copper produced. Figure 1 shows the effects of such variations. For a high value of this coefficient, a single switch point exists. The Alpha technique is cost-minimizing at high wages (or low rates of profits). The Beta technique is cost-minimizing at low wages (or high rates of profits).
Suppose that technical innovations reduce u to 3/2. Then the switch point occurs at the maximum wage. For all positive rates of profits (not exceeding the maximum), the Beta technique is cost-minimizing. At a rate of profits of zero, both techniques (or any linear combination of them) are eligible for adoption by cost-minimizing firms.
A third regime arises when technical innovations reduce u even more. The a technique is cost-minimizing for all feasible rates of profits, including a rate of profits of zero.
4.0 DiscussionSo this example has illustrated that the bifurcation diagram at the top of this previous post can be reflected across a vertical line where the bifurcation occurs. An abstract description of a bifurcation in which a switch point crosses the wage axis does not have a direction, in some sense. Either direction is possible.
The illustrated bifurcation is, in some sense, local. The illustrated phenomenon might occur in what is originally a reswitching example. That is, the bifurcation concerns only what happens around a small rate of profit (or near the maximum wage). It is compatible with wage curves that have a second intersection on the frontier at a higher rate of profits. In such a case, the switch point at the higher rate of profits will remain. But the bifurcation will transform it from a 'perverse' switch point to a 'normal' one.
As I understand it, such a bifurcation of a reswitching will be manifested in the labor market with 'paradoxical' behavior. Suppose the first switch point disappears over the wage axis. Around the second switch point, a comparison of long period (stationary) positions will find a higher wage associated with the adoption of a technique that requires less labor per (net) unit output, for the economy as a whole. But, in the corn industry, a higher wage will be associated with the adoption of a technique that requires more labor per (gross) unit corn produced.
This is just one of those possibilities that demonstrates the Cambridge Capital Controversy is not merely a critique of aggregation, macroeconomics, and the aggregate production function. It has implications for microeconomics, too.