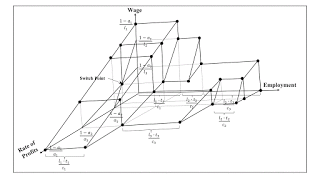
Figure 1: A Labor Demand Function1.0 Introduction I am not sure the above graph works. I could draw three-dimensional graphs in PowerPoint, for models specified with algebra, where relative sizes are indefinite. But, I would need to be able to draw parallel lines, and so on. This post presents a model of extensive rent, with one produced commodity. A labor demand function, for a given rate of profits, graphs real wages versus employment. The resulting function is a non-increasing step function. Net output, in the model, varies with employment. This post was inspired by Exercise 7.5 of Chapter 10 (p. 312) and Section 1 of Chapter 14 (pp. 428-432) of Kurz and Salvadori (1995). I gather one can advance the same sort of argument in a model with intensive rent or with a mixture of
Topics:
Robert Vienneau considers the following as important: Example in Mathematical Economics, Joint Production
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes Double Fluke Cases For Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes The Emergence of Triple Switching and the Rarity of Reswitching Explained
Robert Vienneau writes Recap For A Triple -Switching Example
|
| Figure 1: A Labor Demand Function |
I am not sure the above graph works. I could draw three-dimensional graphs in PowerPoint, for models specified with algebra, where relative sizes are indefinite. But, I would need to be able to draw parallel lines, and so on.
This post presents a model of extensive rent, with one produced commodity. A labor demand function, for a given rate of profits, graphs real wages versus employment. The resulting function is a non-increasing step function. Net output, in the model, varies with employment.
This post was inspired by Exercise 7.5 of Chapter 10 (p. 312) and Section 1 of Chapter 14 (pp. 428-432) of Kurz and Salvadori (1995). I gather one can advance the same sort of argument in a model with intensive rent or with a mixture of intensive and extensive rent. I conclude with some observations about generalizing this approach to models with multiple produced commodities.
2.0 TechnologyLand is in fixed supply in this model. Three types of land exist. I assume tj acres of Type j land are available. Capitalists know of a single process for producing corn on each type of land. Table 1 displays the coefficients of production for each process.
| Inputs | Corn-Producing Processes | ||
| Alpha | Beta | Gamma | |
| Labor | l1 | l2 | l3 |
| Type I Land | c1 | 0 | 0 |
| Type II Land | 0 | c2 | 0 |
| Type III Land | 0 | 0 | c3 |
| Corn | a1 | a2 | a3 |
| Outputs | 1 Bushel Corn | ||
I make a number of assumptions:
- Each process exhibits constant returns to scale.
- All processes require a year to complete and totally consume their capital (seed corn).
- Wages and rent are paid out of the surplus product at the end of the year.
- All parameters (tj, aj, lj, cj) are positive.
- Each input of corn per bushel corn produced, aj, is less than one.
- Without loss of generality, I assume:
(1 - a1)/l1 > (1 - a2)/l2 > (1 - a3)/l3
- In this specific case:
a1 < a3 < a23.0 Price Equations
Prices must be such that, for j = 1, 2, and 3, the following inequality holds:
aj(1 + r) + ljw + cjqj ≥ 1
where w is the wage, r is the rate of profits, and qj is the rent on land of Type j. When the above is a strict equality, the corn-producing process with the given index incurs extra costs and will not be operated.
In a self-sustaining state, the above equation will be met with equality for at least some processes. For almost all feasible levels of employment, the equality will be met with one type of land, known as the marginal land, paying no rent. The marginal land will be partially in use, but some of it will be in excess supply. Other types of land, if any, that pay a rent will be fully used.
4.0 The Choice of TechniqueThe problem becomes to determine the order in which land is cultivated, as employment increases; the marginal land; and the corresponding wage and rents. I take the rate of profits as given in this analysis.
Consider a vertical line (not necessarily just the ones shown) on the wage-rate of profits plane, with employment set to zero. This line should be drawn at a given rate of profits. Three wage curves are drawn on this plane, each for an equality in the above equation, with rent set to zero. Each line connects the maximum wage, (1 - aj)/lj bushels per person-year, with the maximum rate of profits, (1 - aj)/aj, for the corresponding process.
The intersections of the wage curves, on this plane, with the vertical line you have drawn, working downward, establishes an order of types of land. I have given the assumptions such that this order is Type 1, Type 2, and Type 3 land when the rate of profits is zero. At the switch point, Type 2 and Type 3 land are tied in this order. For a somewhat larger rate of profits, the order is Type 1, Type 3, and Type 2.
This is the order in which lands are cultivated as output expands. Accordingly, I have drawn labor demand curves as step functions in planes parallel to the wage-employment plane. The height of these steps are determined by the wage that is paid on the marginal land. The height decreases as the rate of profits increases. The width of each step corresponds to how much employment is needed to fully use that land.
The lands for the steps higher and to the left of any point on the step function for the demand function for labor pay a rent when employment and wages are as at that point, in a self-reproducing equilibrium. The lands for the steps lower and to the right pay no rent and are not farmed. If the point is somewhere on the horizontal portion of a step, that land is marginal. Some of it lies fallow, and it pays no rent.
5.0 The Marginal Productivity of LaborI might as well explain in what sense the wage is equal to the marginal productivity of labor at any point along the demand curve for labor. For the sake of argument, take the rate of profits, r, as fixed. I assume types of land have been re-indexed in order of cultivation, as described above. An ordered pair (L, w) on the labor demand function is either on a horizontal step or a vertical line segment between steps.
First, consider a horizontal step. An increment of labor, ∂L, results in an increased gross output of (∂L/li) bushels of corn. This increased gross output requires an increased input of (∂L ai/li) bushels of seed corn. The increased net output would be the difference between the increment of gross output and the increment of seed corn if these changes occurred at the same moment in time. Either the increased output (and the wage) must be discounted back to the start of the year or the increment in seed corn must be costed up for the end of the year. Adopting the latter alternative, an increment of labor results in a marginal increase in net output of [(∂L/li) - (1 + r)(∂L ai/li)] bushels of corn.
Second, consider a vertical drop. Then, the marginal net product of labor is specified by an interval. In linear models of production, the "equality" of the wage with the marginal product of labor is expressed by an interval bounding the wage:
(1/li) - ai(1 + r)/li ≤ w ≤ (1/li - 1) - ai - 1(1 + r)/li - 1
The marginal product of labor is not a physical quantity, independent of prices. It depends on the rate of profits, an important variable in any model of distribution.
6.0 ConclusionThe above is an exposition of a modern analysis of a special case of Ricardo's theory of extensive rent. Mainstream microeconomics can be viewed, after 1870, as (mostly) an unwarranted extension of Ricardo's theory of rent, especially his theory of intensive rent.
Explaining equilibrium prices and quantities by intersections of well-behaved supply and demand functions makes no sense, in general. In particular, wages and employment cannot be explained by supply and demand functions. The above example fails to illustrate this result.
Two limitations of this example, which do not generalize to a model with multiple produced commodities, perhaps account for this failure. First, no distinction can be drawn in the model between demand for labor in the corn-producing sector and demand for labor in the economy as a whole. Increased employment results in both increased gross and increased net output of corn. It is impossible, in this model, for another process to be adopted in an industrial sector (which does not require land as input) such that less corn is required for gross output in the corn sector, for a greater net output in the economy as a whole.
Second, corn capital and output are homogeneous with one another. Different wage levels may result in the adoption of a different process on (newly) marginal land. But no possibility arises in the model for components of capital to vary in relative price with one another. (Prices must vary for the long-period method to be applied in the analysis of labor demand. But prices, other than wages, cannot vary for (some) conceptions of the neoclassical long-period labor demand function. See Vienneau (2005).)
Oppocher and Steedman's 2015 book expands on these points. I was interested to find out that various mainstream economists had developed a new long-period theory of the firm, in the late 1960s and early 1970s, in which a variation in one price must be compensated for by a variation in other prices.
 Heterodox
Heterodox
