Figure 1: Specialization In A Single Country1.0 Introduction This post considers how the firms in a small open economy will specialize, given prices on international markets and the domestic rate of profits. The example would only be interesting as part of a larger argument, which I have not yet worked out. 2.0 Technology Consider a small, open economy which has a flow-input, point-output technology for producing two consumption goods, corn and linen. Corn is manufactured from inputs of direct labor and steel. Steel and linen are each manufactured from inputs of direct, unassisted labor. Table 1 shows an input-output table for the technology. The technology is thus specified by three coefficients of production, (l1, C, n, l2, C, n, and l1, L, n). Table 1: Example Technology
Topics:
Robert Vienneau considers the following as important: Austrian School Of Economics, Example in Mathematical Economics, international trade
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes Double Fluke Cases For Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes The Emergence of Triple Switching and the Rarity of Reswitching Explained
Robert Vienneau writes Recap For A Triple -Switching Example
|
| Figure 1: Specialization In A Single Country |
This post considers how the firms in a small open economy will specialize, given prices on international markets and the domestic rate of profits. The example would only be interesting as part of a larger argument, which I have not yet worked out.
2.0 TechnologyConsider a small, open economy which has a flow-input, point-output technology for producing two consumption goods, corn and linen. Corn is manufactured from inputs of direct labor and steel. Steel and linen are each manufactured from inputs of direct, unassisted labor. Table 1 shows an input-output table for the technology. The technology is thus specified by three coefficients of production, (l1, C, n, l2, C, n, and l1, L, n).
| Input | Industry | ||
| Steel | Corn | Linen | |
| Labor | l2, C, n Person-Yrs | l1, C, n Person-Yrs | l1, L, n Person-Yrs |
| Steel | 0 | 1 Ton | 0 |
| Linen | 0 | 0 | 0 |
| Corn | 0 | 0 | 0 |
| Output | 1 Ton | 1 Bushel | 1 Square-Yd |
Comparative advantage when the rates of profits is zero in each country is determined by relative ratios of labor embodied in each commodity. The labor value of steel is:
vS, n = l2, C, n
The labor value of corn is the sum of the labor embodied in steel used in producing corn and the direct labor used in producing corn:
vC, n = l1, C, n + l2, C, n
The labor value of linen is:
vL, n = l1, L, n
For a technology in which dated labor inputs extend for a larger number of time periods, finding labor values can require the calculation of sums with a greater number of terms.
3.0 AutarkyIn this section, I assume foreign trade is not possible for the country being analyzed.
Let pC, n, pL, n, and pS, n be the domestic prices of corn, linen, and steel when no foreign trade is possible. Let wn be the wage paid for a person-year of labor, and let rn be the rate of profits. Assume labor is advanced and wages are paid out of the surplus at the end of the year.
Under these assumptions, prices, with labor-commanded as the numeraire, are:
pS, n/wn = l2, C, n = vS, n
pC, n/wn = vC, n + l2, C, nrn
pL, n/wn = l1, L, n = vL, n4.0 Trade in Consumer Goods
Now suppose foreign trade is possible in consumer goods, but not in capital goods. In terms of the example, the firm can trade in corn and linen, but not in steel. Let PC and PL be international prices of corn and linen, respectively.
Two patterns of complete specialization are possible. Suppose the firms in the country want to produce linen (and the required steel), but not corn. Linen is sold on international markets, and corn is purchased. For firms to be unwilling to produce corn, the cost of producing a unit of corn, at the going rate of profits, must exceed the given international price:
[l2, C, n( 1 + rn) + l1, C, n] wn > PC
In a steady state, the cost of producing linen must be equal to its price:
l1, L, nwn = PL
Solving for the wage and substituting, one obtains the following inequality.
PL/PC > l1, L, n/(vC, n + l2, C, nrn)
Or:
PL/PC > pL, n/pC, n
For domestic firms to want to specialize in producing corn, the above inequality is reversed. In words, the country specializes in producing those goods whose international prices exceed autarkic prices.
5.0 Trade in Capital and Consumer GoodsIn this section, I assume that the country can trade steel on international markets, as well as corn and linen.
Suppose the country specializes in producing linen. The following inequalities and equalities must be satisfied in a steady state:
l2, C, nwn > PS
PS ( 1 + rn) + l1, C, nwn > PC
l1, L, nwn = PL
Following my usual practice of solving for wages and substituting, I obtain two inequalities:
PL > (l1, L, n/l2, C, n) PS
PL > (l1, L, n/l1, C, n)[PC - PS ( 1 + rn)]
When the country specializes in corn, the system of inequalities and equalities is modified in a way that I hope is obvious. As above, two conditions characterize prices on international markets. The same is true for when the country specializes in the production of steel. For the country to specialize in linen, it is necessary but not sufficient that the country would have specialized in linen in trade in steel were impossible. This is not so for specialization in corn. For some combinations of international prices, the country will specialize in corn even when the country would not have so specialized when trade in capital goods was not possible. Likewise, a set of prices exists in which the country specializes in steel when the country would have specialized in corn without the possibility of trade in steel.
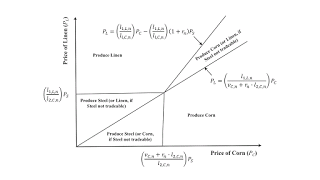
6.0 ConclusionFigure 1, at the head of this post summarizes the analysis. The upward-sloping line extending from the origin divides regions of specialization under the assumption that foreign markets exist for consumer goods, but not for capital goods. When the international prices of corn and linen are in the region above this line, the country specializes in producing linen. When they are in the region below this line, the country specializes in producing corn.
With the possibility of international trade in steel, additional regions appear. If prices of linen and corn are low enough, for a given price of steel, they fall in the rectangle at the lower left in the figure. The country specializes in steel. All of each year's output of steel is sold in foreign trade, and linen and corn are bought with the revenues thereby obtained. A wedge appears in the upper right. If prices are in this region, the country does not specialize in producing linen. It becomes more cost-effective to produce corn, with inputs of steel purchased on international markets.
 Heterodox
Heterodox
