Figure 1: Variation of Prices of Production with Wages and Markups1.0 Introduction This post documents an example in my working paper, The Labor Theory of Value and Sraffa's Standard Commodity with Markup Pricing. 2.0 Technology Consider a simple economy in which corn and ale are each produced from inputs of labor, corn, and ale. Inputs for unit outputs are shown in the columns in Table 1. Obviously, the units of measure should not be taken serious. Inputs are totally used up in the production of outputs. I abstract from the existence of fixed capital, land, and joint production. Table 1: The Technology InputIndustryCornAleLabor1 Person Year1 Person-YearCorn(1/8) Bushels(3/8) BushelsAle(1/16) Pints(1/16) Pints The standard net product consists of (9/16) bushels corn and (3/16) pints
Topics:
Robert Vienneau considers the following as important: Example in Mathematical Economics, Karl Marx
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes Double Fluke Cases For Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes The Emergence of Triple Switching and the Rarity of Reswitching Explained
Robert Vienneau writes Recap For A Triple -Switching Example
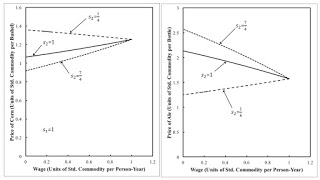
|
| Figure 1: Variation of Prices of Production with Wages and Markups |
This post documents an example in my working paper, The Labor Theory of Value and Sraffa's Standard Commodity with Markup Pricing.
2.0 TechnologyConsider a simple economy in which corn and ale are each produced from inputs of labor, corn, and ale. Inputs for unit outputs are shown in the columns in Table 1. Obviously, the units of measure should not be taken serious. Inputs are totally used up in the production of outputs. I abstract from the existence of fixed capital, land, and joint production.
| Input | Industry | |
| Corn | Ale | |
| Labor | 1 Person Year | 1 Person-Year |
| Corn | (1/8) Bushels | (3/8) Bushels |
| Ale | (1/16) Pints | (1/16) Pints |
The standard net product consists of (9/16) bushels corn and (3/16) pints ale. The Perron-Frobenius root of the Leontief input-output matrix is 1/4. (The other eigenvalue is (-1/16). The maximum rate of profits is 300 per cent. Labor values are (64/51) person years per bushel corn and (80/51) person-years per pint ale.
3.0 Price EquationsEquations for prices of production are:
[(1/8) pcorn + (1/16) pale]( 1 + r̂) + w = pcorn
[(3/8) pcorn + (1/16) pale]( 1 + s2 r̂) + w = pale
(9/16) pcorn + (3/16) pale = 1
I have taken the standard commodity as the numeraire. This allows one to freely move back and forth, when evaluating aggregates, from labor values to monetary units.
The rate of profits in producing corn is 100 r̂ percent, while it is s2 r̂ percent in producing ale. I am assuming there are persistent barriers to entry or some reason why the rate of profits persistently varies between industries. Some economists talk about dual markets. I can also point to John Kenneth Galbraith's The New Industrial State for a contrast of corporations in the planning system and more traditional firms. Anyways, the solution of these equations is:
pcorn = 16 [16 + (1 - s2) r̂]/[204 + (3 + 9 s2) r̂]
pale = 32 [10 - (1 - 3 s2) r̂]/[204 + (3 + 9 s2) r̂]
w = 4 [51 - (9 + 5 s2) r̂ - s2 r̂2]/[204 + (3 + 9 s2) r̂]
These equations show that prices of production vary from labor values when the rate of profits is positive. Furthermore, these are not straight lines, although the curvature is not visually impressive in the figure at the top of this post.
Anyways, here is a question. Suppose labor coefficients happen to be a left-hand eigenvector of the Leontief input-output matrix, a very special case. When prices of production are defined with equal rates of profits across all industries, prices of production are labor values in this special case. (The specification of the numeraire does not matter.) Does this property still hold under the sort of markup pricing which I am assuming?
 Heterodox
Heterodox
