Figure 1: A Production Network I here comment on Baqaee and Farhi (2018). I am still trying to absorb it. I suppose that it is nice that an economist at Harvard is revisiting the Cambridge Capital Controversy (CCC). (Where is Michael Mandler these days?) My major criticism is they do not do what their title claims. That is, their supposed microeconomic foundations are still up in the air. In many CCC examples, technology is specified in terms of fixed-coefficient production processes. Sometimes, more than one process is available for producing a specified commodity. This structure gives rise to a choice of technique. If one wanted, one could formulate a programming problem, in each sector, whose solution is a production function for that sector. This production function would not be
Topics:
Robert Vienneau considers the following as important: Criticisms of Sraffian Economics
This could be interesting, too:
Robert Vienneau writes Brad DeLong, Noah Smith, And Others On The Cambridge Capital Controversy
Robert Vienneau writes “When Economists Are Wrong”
Robert Vienneau writes Some Positions Some Take On Sraffa’s Book
Robert Vienneau writes On Milana’s Purported Solution To The Reswitching Paradox
|
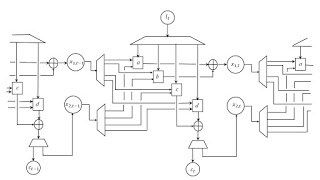
| Figure 1: A Production Network |
I here comment on Baqaee and Farhi (2018). I am still trying to absorb it. I suppose that it is nice that an economist at Harvard is revisiting the Cambridge Capital Controversy (CCC). (Where is Michael Mandler these days?)
My major criticism is they do not do what their title claims. That is, their supposed microeconomic foundations are still up in the air.
In many CCC examples, technology is specified in terms of fixed-coefficient production processes. Sometimes, more than one process is available for producing a specified commodity. This structure gives rise to a choice of technique. If one wanted, one could formulate a programming problem, in each sector, whose solution is a production function for that sector. This production function would not be differentiable everywhere.
Baqaee and Farhi, on the other hand, assume the existence of continuously-differentiable microeconomic production functions in each sector. In this paper, these production functions are specifically Constant Elasticity of Scale (CES) production functions. (As should be the case, their inputs and outputs are specified in physical units, not in price terms.)
I am willing to be convinced that this difference in starting points is a technical matter. Or that Baqaee and Farhi are making some progress towards a more complete framework that will include models of the production of commodities by means of commodities. I have some challenges, however.
There is a theorem whose status I am not sure of. It states that, given a continuously differentiable production function for a commodity that is basic, in the sense of Sraffa, for all techniques, reswitching is not possible. (Stephen Marglin was not the first to offer a proof of this theorem.) Thus, Baqaee and Farhi rule out, by assumption, many (most?) of the reswitching examples and much of the structure in the literature on the CCC. As they note, their assumptions do include an example from Paul Samuelson, in his 1966 "Summing Up" article. That example, had a flow-input, point-output structure, with no commodity basic in any technique.
Second, I gather Baqaee and Farhi think of themselves as starting with microeconomic data that is in principle empirically observable. These would be elasticities of substitution at points on factor demand curves that are chosen at an instance of time. Part of the point of the CCC is to question the existence of factor demand curves, including for intermediate inputs. In a comparison of long period positions, it is an incoherent thought experiment to vary one price at a time. On the other hand, as Han and Schefold have shown, empirical work can be based on given fixed coefficients processes. I think if Baqaee and Farhi were to take this point, they would have to rewrite a lot of their paper, including sections talking about the bias of technical change and macroeconomic elasticities of substitution between factors.
Baqaee and Farhi do have an interesting suggestion for visualizing a production network in logical time. (I've previously presented a less detailed approach from Bidard.) My diagram above is an attempt to expand on Baqaee and Farhi's approach. For each time period, four processes (a, b, c, and d) exist for producing one of two commodities from inputs of labor and those two commodities. The first two processes have the first good as output, and the second two processes produce the second good. The second commodity can be used for consumption, as well as a capital good in the production of either good. This is basically the technology for the examples in Vienneau (2005). The diagram could be simplified by not explicitly showing the demultiplexers and the summations.
References- David Rezza Baqaee and Emmanuel Farhi (2018). The Microeconomic Foundations of Aggregate Production Functions. 26 November.
- Robert L. Vienneau (2005). On Labour Demand and Equilibria of the Firm. Manchester School 73(5): 612-619.
 Heterodox
Heterodox
