Figure 1: Regions for Basis Variables 1.0 Introduction I introduced a new way of visualizing the choice of technique for two-commodity models back in 2005. As far as I know, nobody has taken up this idea. I modify my method slightly by having labor advanced; wages are paid out of the surplus at the end of the year. I cite John Roemer in my paper linked previously. 2.0 Technology Table 1 specifies the technology I use for illustration. Each row lists the inputs needed to produce one unit (ton or bushel) for the indicated industry. As usual, this is a model of circulating capital. Table 1: Example Technology InputIndustryIronCornAlphaBetaLabora0, 1 = 1aα0, 2 ≈ 0.9364aβ0, 2 ≈ 0.6174Irona1, 1 = 9/20aα1, 2 ≈ 0.02602aβ1, 2 ≈ 0.001518Corna2, 1 = 2aα2, 2
Topics:
Robert Vienneau considers the following as important: Example in Mathematical Economics, Labor Markets, Sraffa Effects
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes Double Fluke Cases For Triple-Switching In The Corn-Tractor Model
Robert Vienneau writes The Emergence of Triple Switching and the Rarity of Reswitching Explained
Robert Vienneau writes Recap For A Triple -Switching Example
|
| Figure 1: Regions for Basis Variables |
I introduced a new way of visualizing the choice of technique for two-commodity models back in 2005. As far as I know, nobody has taken up this idea. I modify my method slightly by having labor advanced; wages are paid out of the surplus at the end of the year. I cite John Roemer in my paper linked previously.
2.0 TechnologyTable 1 specifies the technology I use for illustration. Each row lists the inputs needed to produce one unit (ton or bushel) for the indicated industry. As usual, this is a model of circulating capital.
| Input | Industry | ||
| Iron | Corn | ||
| Alpha | Beta | ||
| Labor | a0, 1 = 1 | aα0, 2 ≈ 0.9364 | aβ0, 2 ≈ 0.6174 |
| Iron | a1, 1 = 9/20 | aα1, 2 ≈ 0.02602 | aβ1, 2 ≈ 0.001518 |
| Corn | a2, 1 = 2 | aα2, 2 ≈ 0.1041 | aβ2, 2 ≈ 0.4636 |
For this economy to be reproducible, both iron and corn must be produced. The iron-producing process can be combined with either of the corn-producing processes. Thus, there are two possible techniques, the Alpha and Beta techniques, each of which include the corn-producing process with the corresponding label. (The approach in this post can be extended to include any number of available processes in either industry.)
3.0 A Linear Program for the FirmConsider a firm that starts the year with an inventory of ω1 tons iron and ω2 bushels corn. I take corn as the numeraire. The firm faces a price for iron of p bushels per ton and a wage of w bushels per person years. The managers of the firm must set the value of the following decision variables:
- q1: The tons iron produced with the iron-producing process.
- qα2: The bushels corn produced with the Alpha corn-producing process.
- qβ2: The bushels corn produced with the Beta corn-producing process.
- q3: The value of inventory that the firm carries over unused to the next year.
The firm is constrained by the value of its inventory. Its level of production cannot require it to advance more than the value of its inventory.
The managers of the firm attempt to maximize the increment of value. Their problem can be formulated as a Linear Program (LP). They choose q1, qα2, and qβ2 to maximize:
z = (p - pa1, 1 - a2, 1 - a0, 1w)q1+ (1 - paα1, 2 - aα2, 2 - aα0, 2w)qα2+ (1 - paβ1, 2 - aβ2, 2 - aβ0, 2w)qβ2
Such that:
(pa1, 1 + a2, 1)q1+ (paα1, 2 + aα2, 2)qα2+ (paβ1, 2 + aβ2, 2)qβ2≤ p ω1 + ω2
q1 ≥ 0, qα2 ≥ 0, qβ2 ≥ 0
In solving this LP by the simplex method, it is convenient to introduce the slack variable, q3, to convert the constraint to an equality.
4.0 The Dual LPThe above LP has a dual. It is to choose a non-negative rate of profits so as to minimize the capital charge on the inventory. Constraints are such that the cost of each production process, including a charge for capital, does not fall below the revenue from operating that process. Formally, choose r to minimize:
(p ω1 + ω2) r
Such that:
(pa1, 1 + a2, 1)(1 + r) + a0, 1w ≥ p
(paα1, 2 + aα2, 2)(1 + r) + aα0, 2w ≥ 1
(paβ1, 2 + aβ2, 2)(1 + r) + aβ0, 2w ≥ 1
r ≥ 0
If the primal LP has a solution, so will the dual LP. And the value of the objective functions will be the same, for a solution, for both the primal and dual LP. When a decision variable is positive in a solution to the primal LP, the corresponding constraint is met with equality in the dual LP. Thus, if the solution of the primal LP leads to corn being produced and iron being produced with the Alpha iron-producing process, the economy will be on the wage curve for the Alpha technique. Similar remarks apply to the Beta technique.
| Variable in Basis | Value | When Optimal |
| q1 | (p ω1 + ω2)/(pa1, 1 + a2, 1) | r1 ≥ rα2 |
| r1 ≥ rβ | ||
| c1 ≤ p | ||
| qα2 | (p ω1 + ω2)/(paα1, 2 + aα2, 2) | r1 ≤ rα2 |
| rα2 ≥ rβ2 | ||
| cα2 ≤ 1 | ||
| qβ2 | (p ω1 + ω2)/(paβ1, 2 + aβ2, 2) | r1 ≤ rβ2 |
| rα2 ≤ rβ2 | ||
| cβ2 ≤ 1 | ||
| q3 | p ω1 + ω2 | c1 ≥ p |
| cα2 ≥ 1 | ||
| cβ2 ≥ 1 |
The solution to the primal LP is illustrated by Table 2. In a solution, only basis variables are positive. The table specifies the value of each basis variable, when only it is positive in the solution, and conditions that must hold for it to be in the basis. These conditions are specified in terms of certain variables introduced as abbreviations. The rates of profits in each process are:
r1 = (p - a0, 1w)/(pa1, 1 + a2, 1)
rα2 = (1 - aα0, 2w)/(paα1, 2 + aα2, 2)
rβ2 = (1 - aβ0, 2w)/(paβ1, 2 + aβ2, 2)
The (undiscounted) costs of each process are:
c1 = pa1, 1 + a2, 1 + a0, 1w
cα2 = paα1, 2 + aα2, 2 + aα0, 2w
cβ2 = paβ1, 2 + aβ2, 2 + aβ0, 2wThe conditions for when a decision variable is in the basis are intuitive. Consider the first row. Corn is produced only if the rate of profits made in either of the iron-producing processes does not exceed the rate of profits made in the corn producing process. Furthermore, the (undiscounted) cost of producing a bushel corn must not exceed the revenue made from selling corn. 6.0 Visualization
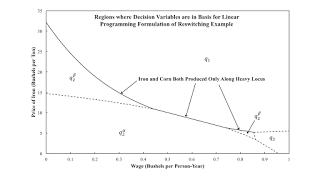
The solution to the primal LP, in a two-commodity example, is easily visualized. Figure 1 partitions the space formed from the price of iron and the wage. A single decision variable enters the basis inside each region in the figure, and that region is labeled by that decision variable. On the boundaries, a solution to the LP can be formed from a linear combination of decision variables. Iron and corn must be both produced for the economy to be self-sustaining. Firms are willing to produce both only if prices lie along the heavy locus. The figure shows that this is a reswitching example. The Beta technique is adopted at low and high wages, while the Alpha technique is used at intermediate wages. The figure also illustrates that the wage cannot exceed a maximum.
7.0 ConclusionIf you think about it, the above is a derivation of the usual method of analyzing the choice of technique by constructing the outer frontier of the wage curves for all available techniques. It is not restricted to a two-commodity example, although the diagram is so restricted. The proof follows from duality theory in linear programming. The graph illustrates that equilibrium prices must vary with the wage.
I remain puzzled about why mainstream economists continue to teach that, under the ideal assumptions of free competition, wages and employment are determined by the interaction of supply and demand in labor markets.
 Heterodox
Heterodox
