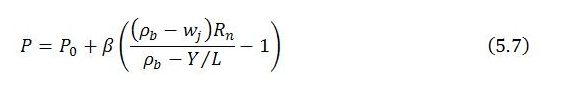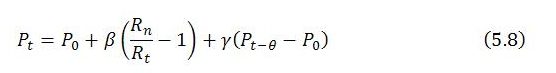Macro Dynamics with a Job Guarantee – Part 5: Price Level

by
peterc
My articles My booksMy videos
Follow on:Twitter

So far, in considering a simplified economy with a job guarantee, the focus has been on the demand-determined behavior of output and employment. Prices, in this exercise, have simply been taken as given on the grounds that they are not causally significant in the process. This approach does not require prices to remain constant, though, for given supply conditions, they may well do so over a fairly wide range of output for reasons to be discussed. Nor does it require that prices are necessarily unrelated to output; only that the direction of causation in any aggregate relationship between the two mostly runs from output to prices rather than the other way round. But once attention turns to the issue of price stability, which is of considerable interest to job-guarantee proponents, it becomes relevant to entertain a possible short-run relationship between output and prices. This will provide a basis for identifying potentially price-stabilizing aspects of a job guarantee in the next part of the series.

 
Normal pricing and planned margins of spare capacity

A prevalence of cost-based or normal pricing in much of the economy means that prices can remain stable amid fluctuations in demand so long as supply conditions remain unchanged. Normally, the labor force and the economy’s productive capacity are less than fully utilized, and average variable costs are constant (or even falling) for levels of output below full capacity. These features are not by accident, but by design. Spare capacity is intentionally maintained by for-profit firms as a means of accommodating peak demand rather than risk losing market share to rivals. In public-sector and not-for-profit production, planned margins of spare capacity still give flexibility in catering to varying levels of demand. The flexibility built in to production is especially clear in the long run when there is time to adapt productive capacity to persistent trends in demand through investment or disinvestment, innovate in the development of alternative resources, free up a portion of the labor force for new activities through ongoing improvements in productivity, and train extra workers for roles that temporarily face supply constraints. But it is also largely true in the short run because of the ability to vary the rate at which existing capacity is utilized.

Even so, if demand happens to fluctuate outside the range that can readily be accommodated by variations in the level of production, inflationary or deflationary forces can develop.

 
Limits to price stability

In broad terms, price pressures can originate from the demand or the supply side of the economy. On the demand side, spending that outstrips or falls far short of existing productivity capacity can generate demand-pull inflation or deflation. On the supply side, shortages of key resources or categories of workers can result in bottlenecks even while there is spare plant and equipment. In addition, variations in productivity, wages, the average pricing markup, taxes on production, interest rates and indexation policies can all affect prices through cost channels.

In practice it is not always easy to distinguish supply-side and demand-side influences on the price level, but conceptually it can be helpful to keep them separate.

 
Supply-side influences on the price level

To isolate supply-side factors, suppose there is some level of output Y_n at which demand is neither exerting inflationary nor deflationary pressure on the economy. In reality, there is likely to be a range of output for which this is true, but for simplicity the particular output Y_n will be taken as a reference point. We can think of a demand-induced change in the price level as becoming increasingly likely the further from Y_n the economy moves. Through suitable choice of functional form, it will still be possible to characterize the price level as nearly impervious to demand near Y_n while being sensitive beyond that range.

From a Keynesian or Kaleckian standpoint, and within the model itself, there is no inherent tendency for the economy to move to Y_n unless this particular level of output just so happens to coincide with the steady-state level Y*. In general, this need not be the case. The demand-determined level of output that eliminates unplanned investment (meaning unintended inventory buildup or depletion) need not be the level of output at which demand pressures on the price level are completely absent. The steady-state level of output depends on the level of autonomous demand and the marginal propensity to leak to taxes, saving and imports. In an economy with a job guarantee, it also depends on sectoral productivities and the level of total employment (as outlined earlier in the series). Although it is the case that a steady-state level of output will result in a stable level of prices, since the steady state is predicated on both stable demand and stable supply conditions, this level of prices will differ from the price level associated with Y_n to an extent that depends on the level of demand that underpins output Y*.

In general, the price level can be decomposed into the aggregate markup over wages (m), the average wage (w) and productivity (in the model, sector b productivity ρ_b):

In the special case where output happens to equal Y_n, the price level will solely reflect supply conditions. In the short run, these conditions can be regarded as exogenous:

The supply-determined P₀ reflects the particular markup, average wage and productivity that would apply at output Y_n:

The price level itself will differ from this exogenous level P₀ to the extent that demand conditions cause the realized values of the markup, average wage and productivity to differ from the values they would take at output Y_n.

 
Demand-side influences on the price level

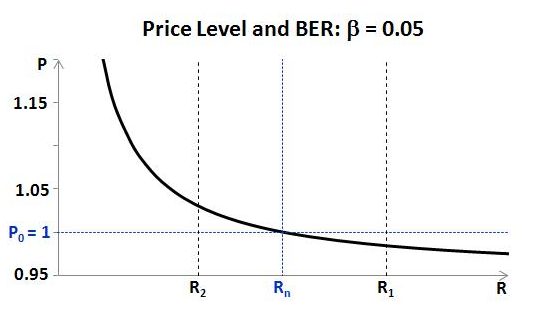
To allow for the influence of demand on prices, it is convenient to choose some index of the state of demand. In an economy with a job guarantee, output Y_n will be associated with a particular ‘buffer employment ratio’. The buffer employment ratio is the proportion of total employment actually located in sector j (the job-guarantee sector). In terms of the model, it is L_j / L. This ratio shrinks with rising demand. The particular buffer employment ratio that corresponds to output Y_n can be denoted R_n. Although R_n is likely to be influenced by the trajectory of demand in the long run, in a short-run setting it can reasonably be assumed constant, reflecting given structural and institutional factors. Whenever R falls below R_n, there will be a degree of demand-side inflationary pressure. Conversely, when R rises above R_n, there will be risk of deflation.

 
Short-run relationship between prices and the buffer employment ratio

The following price equation allows for both cost-push and demand-pull influences:

The exogenous supply-determined component of the price level P₀ has already been encountered. Demand affects prices through the second term, with the price level inversely related to the buffer employment ratio R. The positive parameter β indicates the sensitivity of the price level to demand pressures as expressed through variations in R.

It was established in the previous part of the series that, for given values of the parameters and exogenous variables, the model economy converges on a steady state no matter what the starting point. In a steady state, the buffer employment ratio is stable at R = R*. This makes all terms in (5.4) constant, including the price level, which will be at its steady-state level P*. The steady-state price level will differ from P₀ to the extent that demand-side price pressures are operative.

In the special case where the economy’s steady state coincides with Y_n, the buffer employment ratio stabilizes at R = R_n, and the steady-state price level settles at the purely supply-determined P₀.

Whether the economy is in or out of the steady state, the price level according to (5.4) will be a function of the buffer employment ratio. Provided the value chosen for β is small, the price level will be insensitive to demand for buffer employment ratios near R_n. This is illustrated below for a couple of different values of β.

The functional form of (5.4) is chosen on the basis that prices are less likely to deflate significantly below P₀ than to inflate above P₀. If wages and prices were fully flexible in both directions, this feature of the price equation might be less appropriate. Under full wage and price flexibility, deflation might well be more explosive (and more calamitous) than inflation due to the impact of deflation on debtors and the flow-on effects to the economy as a whole. In reality, though, various institutional factors – including minimum-wage legislation and a prevalence of contracts denominated in nominal terms – mean that prices are less prone to fall much below P₀ in a downturn than they are to rise significantly above it during an inflationary episode. In an economy with a job guarantee, the job-guarantee mechanism itself would play a significant role in limiting deflation.

 
Short-run relationship between prices and output

The inverse relationship between the price level and buffer employment ratio implies a corresponding relationship between the price level and total output.

This relationship can be made explicit starting from the definition of the buffer employment ratio:

The first step in (5.5) recalls the definition of the buffer employment ratio as sector j employment divided by total employment (R = L_j / L). The next step follows because employment in sector j equals the sector’s income divided by its wage (that is, L_j = Y_j / w_j). The last step follows because, as discussed in part 1 of the series, sector j income is a variable fraction ϕj of total income (so that Y_j = ϕjY), where ϕ is the share of wages in job-guarantee spending and j is the share of job-guarantee spending in total income.

Recalling from part 2 that j = qρ_bL/Y – q and q = (w_j / ϕ) / (ρ_b – w_j), the expression for the buffer employment ratio can be written

Substituting this expression for R into (5.4) gives

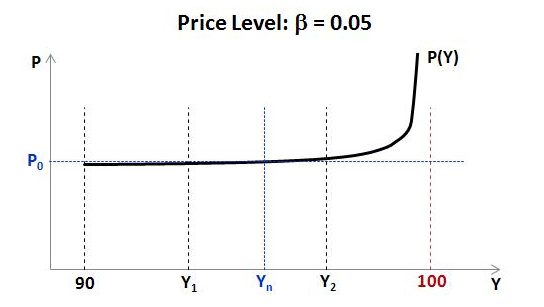
This short-run relationship between the price level and total output is illustrated below for a couple of values of β. In each example, Y_max = 100 and P₀ = 1.

For small values of β, the price level is roughly stable for output in the range (Y₁, Y₂), with price pressures escalating in the vicinity of maximum possible output.

 
Behavior of the price level over time

When considering price behavior over time, it may be relevant to allow a role for expectations in addition to cost-push and demand-pull factors. In preceding sections, any impact from expectations was implicitly subsumed in supply and demand conditions. For example, to the extent that public sector pay may have been influenced by the expected behavior of prices, the effect of this was allowed simply to manifest in the wages and associated expenditures that flowed from that perception.

The following price equation, which modifies (5.4), allows for cost-push and demand-pull influences as well as a role for expectations. In keeping with the emphasis on cost-based or normal pricing, it is supposed that price expectations are influenced by recent variations in the price level relative to the supply-determined P₀.

The time subscripts indicate points in continuous time. Expectations influence the price level through the final term. Any difference between the price level observed at time t – θ and P₀ feeds into the price level of time t to an extent that depends on the nonnegative parameter γ.

Earlier it was observed that, within the model, the price level is stable when the economy is in a steady state but that P* will differ from P₀ when Y* differs from Y_n. This remains true under (5.8) provided γ < 1.

To verify this, suppose that autonomous demand A and the marginal propensity to leak α remain constant for some time such that the economy reaches steady-state output Y = Y* but not necessarily Y_n. The steady-state price level can be found by setting P_t = P_t–θ = P* in (5.8) and solving for P*. The result is

In a steady state, R is stable at R* and the second term in (5.9) represents the sum of a convergent series if γ < 1. Provided this condition is met, the price level converges on a level that depends on the supply-determined P₀, the state of demand (R_n / R*), and the parameters β and γ.

If the steady-state level of output happens to coincide with Y_n, then R* = R_n and the second term in (5.9) vanishes. In that event, the price level converges on P₀. The convergence of P to P₀ is fast for small γ and slow for γ close to 1.

 
Related reading

A few academic papers by Modern Monetary Theorists are particularly relevant to concepts touched on in the post. A 1998 paper by Bill Mitchell on the job guarantee introduces the notion of a ‘non-accelerating inflation buffer employment ratio’ (NAIBER) as an analogue to the non-accelerating inflation rate of unemployment (NAIRU) commonly applied to economies without job guarantees. The level of output Y_n and corresponding buffer employment ratio R_n, employed in the present post as conceptual devices, are based on this idea but relate to the price level rather than the inflation rate.

The Buffer Stock Employment Model and the NAIRU: The Path to Full Employment

The likelihood that Y_n would be influenced by the economy’s demand history was noted in the post. Hysteresis as it relates to unemployment in economies without job guarantees is analyzed by Bill Mitchell in:

The NAIRU, Structural Imbalance and the Macroequilibrium Unemployment Rate

A consideration of factors likely to affect the price level, including a discussion of the approach adopted in the Fair Model, is included in Scott Fullwiler’s simulation study of the job guarantee (pages 10-12 are especially relevant to the topic of the present post).

Macroeconomic Stabilization Through an Employer of Last Resort