by Variable selection — not about having a ‘good fit’ Which independent variables should be included in the equation? The goal is a “good fit” … How can a good fit be recognized? A popular measure for the satisfactoriness of a regression is the coefficient of determination, R2. If this number is large, it is said, the regression gives a good fit … Nothing about R2 supports these claims. This statistic is best regarded as characterizing the geometric shape of the regression points and not much more. The central difficulty with R2 for social scientists is that the independent variables are not subject to experimental manipulation. In some samples, they vary widely, producing large variance; in other cases, the observations are more tightly grouped and there is Little dispersion. The variances are a function of the sample, not of the underlying relationship. Hence they cannot have any real connection to the “strength” of the relationship as social scientists ordinarily use the term, i. e., as a measure of how much effect a given change in independent variable has on the dependent variable … Thus “maximizing R2” cannot be a reasonable procedure for arriving at a strong relationship. It neither measures causal power nor is comparable across samples … “Explaining variance” is not what social science is about.
Topics:
Lars Pålsson Syll considers the following as important:
This could be interesting, too:
Robert Vienneau writes Austrian Capital Theory And Triple-Switching In The Corn-Tractor Model
Mike Norman writes The Accursed Tariffs — NeilW
Mike Norman writes IRS has agreed to share migrants’ tax information with ICE
Mike Norman writes Trump’s “Liberation Day”: Another PR Gag, or Global Reorientation Turning Point? — Simplicius
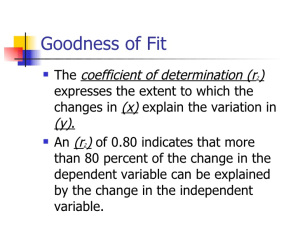Which independent variables should be included in the equation? The goal is a “good fit” … How can a good fit be recognized? A popular measure for the satisfactoriness of a regression is the coefficient of determination, R2. If this number is large, it is said, the regression gives a good fit …
Nothing about R2 supports these claims. This statistic is best regarded as characterizing the geometric shape of the regression points and not much more.
The central difficulty with R2 for social scientists is that the independent variables are not subject to experimental manipulation. In some samples, they vary widely, producing large variance; in other cases, the observations are more tightly grouped and there is Little dispersion. The variances are a function of the sample, not of the underlying relationship. Hence they cannot have any real connection to the “strength” of the relationship as social scientists ordinarily use the term, i. e., as a measure of how much effect a given change in independent variable has on the dependent variable …
Thus “maximizing R2” cannot be a reasonable procedure for arriving at a strong relationship. It neither measures causal power nor is comparable across samples … “Explaining variance” is not what social science is about.